Lissajous Curves

Lissajous figures were developed by a French mathematician in 1857 to visually explore the nature of sound. He taped mirrors on two vibrating tuning forks, bounced light-beams off them and projected the intersecting patterns of harmonic vibrations on the wall.

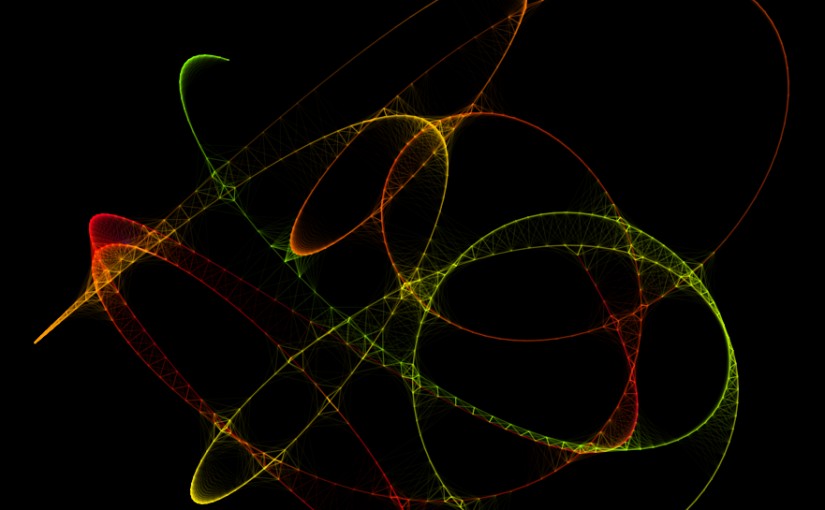
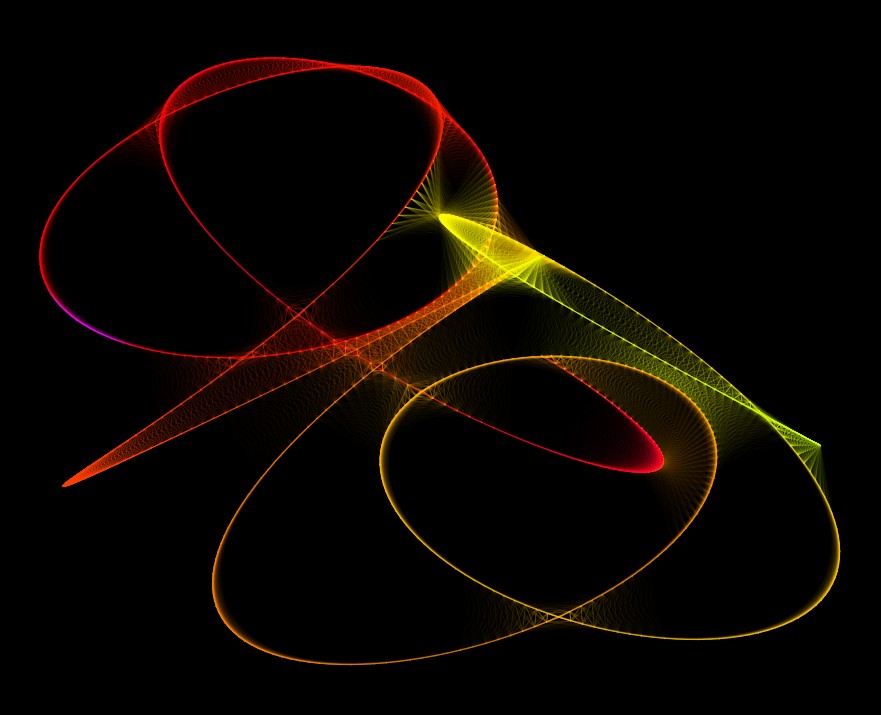
This can be done with code as well by tweaking sine waves, the building blocks of sound and vibration, to emulate a pair of virtual tuning forks using the following formula:

This widget explores Lissajous shapes with code. You can tweak the formula with the sliders on the upper right and save any image you create. The widget cheats a little in the name of artistic license and visualization by connecting points of the curves and extends the formula a bit with additional parameters in lieu of fiddling with actual tuning forks.